



Paul Chodas (NASA/JPL NEO Program Office)
Several months after the discovery of 2015 PDC, the probability of impact in 2022 will have risen to a concerning level. Even if the asteroid is more likely to miss our planet than to hit, mission designers will be asked to study the possibility of deflecting the asteroid, just in case it really is necessary. Arguably the fastest and simplest way to deflect an asteroid is to use a Kinetic Impactor (KI) to hit the asteroid and deflect it off course. Although this app was not designed to be an operational tool for developing such a mission design, it could be used to answer some of the basic questions about deflecting this asteroid.
The following is a quick detailed tour of the app applied to the 2015 PDC scenario object:
Start the app. This initial version starts up with the 2015 PDC scenario object already selected. It also starts up in Delta-V Mode (indicated by orange text on the tab in the upper left), and with the Time of Deflection parameter (D) in the upper left set to 1096 days (3 years) before impact. This app does not use calendar dates: the times are relative to impact, which means to move the deflection time forward, you decrease D. For this quick tour, we'll assume that you have selected "days" as your unit of time for D, and the Advanced Mode and Tips boxes (bottom of the Main Panel) are unchecked.
The Orbit Panel in the lower left shows the orbits of the Object and the Earth, along with their positions at time D (colored dots) and at the impact time (black dot). (There is a blue dot for the Earth, but it happens to be hidden behind the black Impact point at start-up because D is an exact number of years; essentially, the Earth is at the Impact point exactly 3 revolutions before the impact.)
Advance the time by clicking the left-pointing arrow to the left of the D value, and you'll see the how the positions of both the Object and the Earth change. You can advance the time (decrease D) all the way to 1 day before impact and see the positions converge onto the impact point.
The Plot Panel (upper right) defaults to showing a full 30-year plot of the object's Distance from Earth in Astronomical Units (AU). The AU is the mean Earth-Sun distance, or 149,597,870.70 kilometers. Impact occurs at the right edge of the plot, where the distance goes to essentially zero. Notice the close approach to Earth 7.3 years before impact - that's when the 2015 PDC gets discovered in our scenario. We've loaded a full 30 years of orbital dynamics into the app so that longer warning-time variations of the scenario can also be studied. This object might have been discovered at the close approach 26 years before impact, but that would have been in the year 1996, and the Spaceguard asteroid survey program had not yet begun.
In the box above the plot, click on Dist. From Sun. The app switches to the much more regular pattern of the object's heliocentric distance. Its closest point to the Sun (perihelion) is inside 1 AU, and farthest point (aphelion) beyond 2.5 AU. Because of Kepler's Third Law, the NEO spends most of its time traveling the outer reaches of its orbit, and very little time around perihelion. Note that the NEO makes only about 3 orbits of the Sun during the 7.3 years between discovery and impact.
In the B-Plane Panel (lower right), notice the green dot. That represents the point where the trajectory is taking the asteroid, basically the view from the approaching asteroid showing where it is headed. When the green dot is inside the solid red circle, the asteroid is on an impact trajectory (also indicated by the word IMPACT in the Values Panel to the left). The solid red circle denotes the Earth's "capture cross section" in the B-Plane; it's larger than the actual size of the Earth (blue circle) because it takes into account the focusing of trajectories due to the Earth's gravity. For the PDC15 orbit, the radius of the capture cross section (the "capture radius") is 1.42 times the Earth's radius.
Let's see what it takes to deflect PDC15 off the Earth. First, reset the app to the defaults by clicking Reset in the Main Panel. Leave D set at the default 1096 days. Apply some along-track velocity change ΔVA by clicking the right-pointing arrow next to its slider; the text box to the right indicates the velocity change. As you increase ΔVA, you'll notice the green dot moving upwards in the b-plane. At a ΔVA of about 18 mm/s, the trajectory will move outside the solid red circle and impact will be averted. A miss distance of 0.02 Re is shown in place of the word "IMPACT" in the Values Panel.
Try this again, but now with a different component. Reset the app by clicking Reset. Apply a negative cross-track velocity change by decreasing ΔVC by clicking its left-pointing arrow. (Notice that the default stepsize is larger for this component). You will need to set ΔVC to about -120 mm/s to move the trajectory outside the solid red circle. A lot more ΔV is needed if you apply it in the cross-track direction. ΔVN is even less effective at moving the trajectory. Along-track is clearly the best direction to choose when deflecting an asteroid this far ahead of impact, and not just for this object, but in general.
Reset the app again, and in the Plot Panel, click ΔVA Sensitivity in the menu box above the plot. The app displays a handy sensitivity graph showing how much deflection you get in the b-plane for each mm/s of ΔVA velocity change you apply, as a function of Time of Deflection D. Moving the time D closer to one of the peaks in this plot should give you more deflection for a given ΔV. Since there's only 7 years to impact in our scenario, there are only two peaks to choose from. Let's investigate deflecting at the peak at about 5 years before impact, even though that's only 2 years away and it might not be possible to get a KI spacecraft built, launched and hitting the asteroid in only 2 years.
Zoom in on the ΔVA Sensitivity plot by dragging horizontally in the vicinity of the peak at about 5 years. As you drag, the app grays out the portion of the plot you'll be zooming in on. Once you've zoomed in, it's easier to see the peak time. Point at the peak and click: that sets the Time of Deflection D to the time of the peak, which should be about 1761 days. (Or, you could just type 1761 into the Time of Deflection textbox.) Switch the plot to Dist. from Sun and notice how the peak in ΔVA deflection effectiveness correlates with distance from the Sun.
Back in the Main Panel, increment ΔVA as before, by repeatedly clicking the right arrow next to its slider. You'll see that at this new deflection time, it takes only about 6 mm/s of ΔVA velocity change to deflect the trajectory off the Earth. Clearly, a Deflection Time of 1761 days was a better time to deflect the asteroid, as the sensitivity plot indicated.
In the B-Plane Panel, notice that the trajectory has deflected mostly in the vertical direction: the ζ (zeta) component changes the most. In general, deflections have their greatest effect in the zeta direction. That's because zeta is defined to lie along the projection of the object's orbit into the b-plane, and the most significant effect of deflections generally is to move the object along its orbit. Changing the along-track velocity of the asteroid changes its orbital energy, which affects the rate of motion along its orbit. In particular, a positive ΔVA adds energy to the orbit, which lengthens the orbital period slightly, and that makes the asteroid arrive slightly later at the intersection point with the Earth's orbit.
Could a Kinetic Impactor (KI) interceptor mission be launched to impart at least 6 mm/s of velocity change in the ΔVA direction at this deflection time, using existing launch vehicles? You can answer that question by switching the app to Intercept Mode. Click the tab for that mode at the top of the Main Panel. The Main Panel has a new look in this mode, and you're going to have to specify some different parameters. But the Time of Deflection (D) remains the same: leave it set at 1761 days for now.
Instead of ΔV inputs, you now have inputs that allow you to design a Kinetic Impactor mission, and the app will compute the ΔV that your interceptor will apply to the asteroid. The size of the ΔV will depend on the physical properties of the object, and you need to enter these in the bottom part of the Main Panel. The most important parameter is the object's Mass, but you don't specify that parameter directly. Instead, you specify the object's mean diameter and bulk density and the app computes the Mass for you.
How big is 2015 PDC? At this stage, we won't really know, since the asteroid remains too far away to observe with radar, and we probably won't have enough time to send out a precursor mission to characterize its physical properties. All we have is an estimate of the object's absolute magnitude (intrinsic brightness), H = 21.34. If we just assume an average asteroid albedo (reflectivity) of 14%, this corresponds to a mean diameter for 2015 PDC of about 200 meters. So, for now, enter a Diameter value of 0.20 km.
We know even less about the Density of 2015 PDC. We'll just assume a typical asteroid Density: 1.5 g/cm3, which is representative of a porous rock rubble pile. The app takes your mean diameter and density values and automatically computes the mass to be 6.283e+9 kg (over 6 million metric tons), which it reports near the bottom of the Main Panel. Higher densities of 3.0 g/cm3 (for dense rock), or even 8.0 g/cm3 (for a metallic object) are certainly possible, but less likely.
The top half of the Main Panel is where you define your KI interceptor mission. The Transfer Time (L to D) is the length of time between Launch of the spacecraft and the time it hits the asteroid (Deflection); it's initially set to 400 days. Below it are your selections for the Launch Vehicle and the Number of Launches, initially set to a single launch of the Atlas V 551. Over in the Plot Panel notice that there's now a dotted line highlighted in blue and labeled L to indicate the Launch time (you may have to Reset zoom to see it). The Transfer time is the time between the blue line and the yellow line.
In the Orbit Panel, notice that there's now a green dot indicating the position of the Earth at Launch, and the app has computed the trajectory that takes your KI spacecraft from green Launch point to red Deflection point, shown as the dashed green line. In the lower right corner of the panel, the app tells us that the Interceptor would make 0.87 orbits about the Sun from Launch to Deflection. The app solves something called the Lambert Problem to find this trajectory, but it won't always be able to find a transfer trajectory satisfying your inputs and the constraints of the launch vehicle. There are many combinations of D, Transfer Time and Launch Vehicle for which the app will not be able to find a transfer trajectory. There will be no dashed green orbit for those cases.
In the Main Panel, below the Launch Vehicle line, the app reports the "Mass Delivered to Object", which is the maximum mass that can be launched onto this trajectory by your launch vehicle. For D = 1761, L-to-D = 400 days, and a single Atlas V, the maximum mass of the KI spacecraft is computed to be 2476.5 kg. The app requires the spacecraft mass to be at least 500 kg. If your launch vehicle can't launch at least this amount of mass onto the required trajectory, or if a trajectory cannot be found, the app displays "NONE" as the Mass Delivered to Object and you don't have a viable mission.
In the B-Plane panel you probably noticed that the deflected trajectory (the green dot) has moved downwards relative to the original undeflected trajectory (the red dot). The deflection went in the direction opposite to what we saw earlier, which is unfortunate since the shortest way off the Earth in this plot is upwards. The reason for going downwards is that ΔVA is now negative, not positive as before. Unfortunately, you can't just specify the direction for your applied delta-V, it's determined by the transfer trajectory. The app calculates the velocity vector of the spacecraft relative to the object at deflection, and then computes the delta-V by assuming that all of the momentum of the spacecraft interceptor is transferred to the object.
The magnitude of the delta-V also depends on another parameter, the momentum multiplier Beta. This accounts for the extra momentum imparted by the ejecta emitted by the impact. In practice it's unlikely we'll be able to predict the average direction the ejecta will follow. For simplicity, the app assumes the net effect of the ejecta is to add some momentum along the relative velocity vector. The app defaults to a conservative Beta value of 1, which corresponds to no ejecta at all. But you can change Beta to see that larger values will produce greater deflections. For now, leave Beta at 1.
In the Values Panel notice the ΔV your KI spacecraft applied to the object, a total of 1.79 mm/s, and as mentioned earlier, the ΔVA component is negative, -1.455 mm/s, which means that even though you may think from the Orbit diagram that the interceptor is hitting the Object from behind, and therefore applying a positive ΔVA, that's not the case. The asteroid is in a larger orbit than the interceptor and will be moving faster than the spacecraft at the deflection point. The asteroid effectively runs over the interceptor, which means the interceptor hits the asteroid on its front hemisphere, not the back. That's why ΔVA is negative.
The easiest deflection, by far, is to launch the interceptor into the object's path and let the NEO run into it, removing energy from the asteroid's orbit. That means the easiest deflections move the trajectory downwards in b-plane zeta, not upwards. Even though the shortest way off the Earth requires increasing zeta, orbital dynamics force us to decrease zeta, and take the long way off the Earth. This preference for negative ΔVA deflections is related to the fact that our scenario object has an orbital semi-major axis larger than 1 AU; 90% of Potentially Hazardous Asteroids fall into this category.
Consider the following image, which maps the features of the Earth surface into b-plane coordinates for PDC15. (This is not a standard projection: the curvature of the potential trajectories produces considerable distortion towards the edges.) You can imagine this image pasted onto the b-plane panel of the app, fitting inside the red circle. The current nominal impact point lies in the mid-Pacific not too far from Hawaii. The vertical red line is the "risk corridor" of constant xi, along which deflections will move the impact point. Downward deflections in zeta will take the impact point across the Philippines, S. China Sea, S.E. Asia, Myanmar, Bangladesh, India, Pakistan, Afghanistan and Iran before it moves off the Earth entirely.
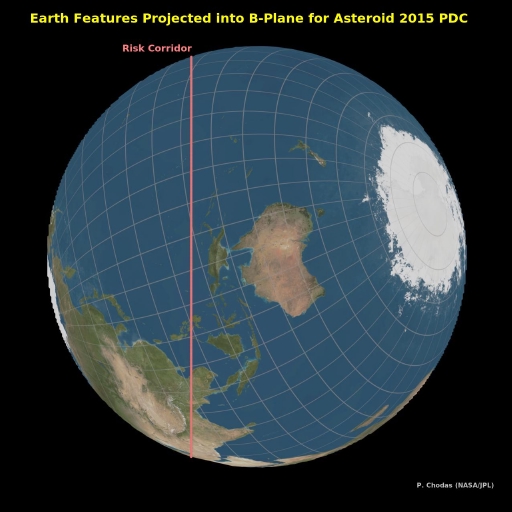
OK, let's accept that we have to move downwards in the b-plane, and see if we can get larger deflections by changing the interceptor trajectory. Start decreasing the Time of Deflection (D) by repeatedly clicking the left-pointing arrow next to its slider. The Total ΔV reaches a maximum of about 14 mm/s at around D = 1711 days. Even though this is no longer at the peak of ΔVA sensitivity, the deflection geometry is more favorable. Change the Transfer Time (L to D) slightly, to 390 days and you get 14.2 mm/s. But this is about the most deflection you can get by changing the trajectory, and you're still a long way from moving the trajectory off the Earth.
An easy way to increase the size of a deflection is to send more interceptors. For simplicity, the app assumes that all of them launch at the same time, follow the same transfer trajectory, and deflect the object at the same time. Change to two launches of the Atlas V and you'll see the deflection double, but it still won't be enough to move the trajectory completely off the Earth (unless Beta is greater than 1). You need a total of about 33 mm/s to deflect off the Earth. Change the Vehicle to the more capable Delta IV Heavy, however, and you can move the trajectory off the Earth with 2 launches, even with a Beta of 1.
Could we actually implement this KI mission? The launch would have to occur around 1711 + 390 = 2101 days before impact, which puts it in late 2016, only about 16 or 17 months away! Suppose it's not possible to build the spacecraft and get them to the launch pad in time. Is there a later launch opportunity?
There are a lot of combinations of L and L-to-D to explore when looking for deflection missions. The app helps you navigate this landscape by providing you with a deflection map. To see it, click the Deflection Map button at the bottom of the page. Using color coding, the map shows the relative size of the b-plane deflection as a function of D and L-to-D; the redder the color, the greater the deflection. To move to deflections earlier than shown on this map (to values of D larger than 2800 days), click the "Earlier' button. But since the object is discovered about 2700 days before impact, there is no need to go beyond the first page of the map. The mission converged to lies in the yellow region at about D = 1700, L-to-D = 400. Let's try the little island of color in the upper right, centered at D = 910 and L-to-D = 200. First, get back to the main screen by clicking Close.
Keep the launch at 2 Delta IV Heavies and Beta at 1. Enter D = 910 days and L-to-D = 200 days, which is near the optimal point in the little island of favorable late launches we mentioned above. Two Delta IV Heavy launches are not enough to move the trajectory off the Earth: now we need 3, giving a total of 37 mm/s of ΔV. The spacecraft designers are happy that you are launching later (1110 days before impact, or Aug. 2019), but now the deflection also occurs later, and you need more delta-V to move the trajectory off the Earth.
Recall that the physical properties of the scenario object are very uncertain. Suppose the brightness measurements were a little off, and the absolute magnitude is more like H = 21.1. And suppose the actual albedo were a little darker than average, say around 7%. That would increase our estimate for the size of the object to about 300 meters. Enter a Diameter of 0.30 km, and see how the Mass goes up dramatically and the resulting deflection falls to about 11 mm/s. Now it would take 10 Delta IV Heavy launches to move the trajectory off the Earth, assuming a Beta of 1. Clearly it would be very important to characterize the physical properties of the scenario object as early and as precisely as possible. Sending a precursor characterization mission, for example, would help enormously in simplifying deflection mission planning.
Finally, recall from the scenario introductory paragraph that the scenario time when we would be doing these initial mission designs is only several months after 2015 PDC's discovery, perhaps in late 2015 or late 2016. The orbit of 2015 PDC will still be quite uncertain, even into mid-2016. That means the position of the trajectory in the b-plane will still be very uncertain in the vertical (zeta) direction. The PDC15 trajectory we've been using so far is simply the best estimate as of early 2016. We probably won't be able to accurately estimate the true zeta position of the trajectory until we've accumulated sufficient tracking observations, perhaps by early 2017. Not until the scenario reaches that point will we know even roughly how much we need to move the trajectory